Half Range Fourier Series Solved Examples Pdf

Full range fourier series various forms of the fourier series 3.

Half range fourier series solved examples pdf. T f 3 t 2 the point is that all three periodic functions f 1 t f 2 t f 3 t will give rise to a different fourier series but all will represent the function f t t2 over 0 t π. Half range fourier series. Half range fourier sine or cosine series a half range fourier sine or cosine series is a series in which only sine terms or only cosine terms are. Even and odd extensions 14 1 even and odd functions even.

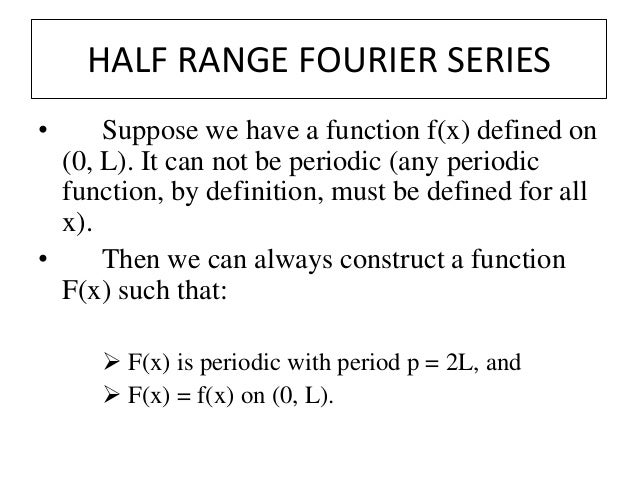
Fourier series obtained by extending functions in this sort of way are often referred to as half. Some of these problems can be solved by use of fourier series see problem 13 24. F x is neither odd or even in this case both a n and b n are non zero and we must use the formulae for the full range. However if f x is discontinuous at this value of x then the series converges to a value that is half way between the two possible function values f x x fourier series converges to half way point vertical jump discontinuity in the function represented toc jj ii j.

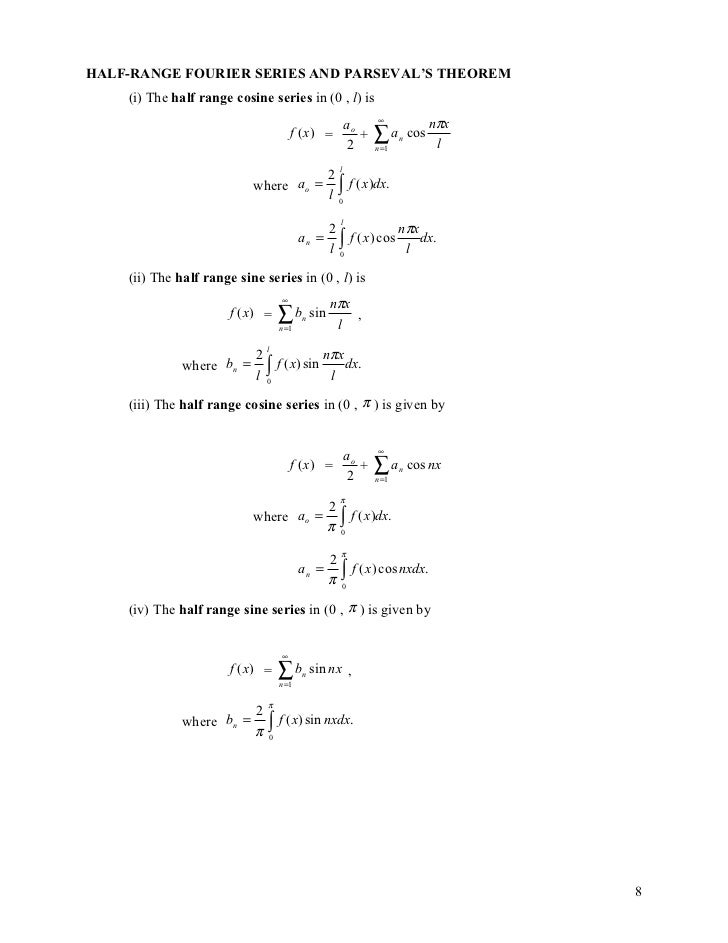
If a function is defined over half the range say 0 to l instead of the full range from l to l it may be expanded in a series of sine terms only or of cosine terms only the series produced is then called a half range fourier series. 1 are given by the standard result for the half range fourier cosine series. Fourier series and integrals 4 1 fourier series for periodic functions this section explains three fourier series.

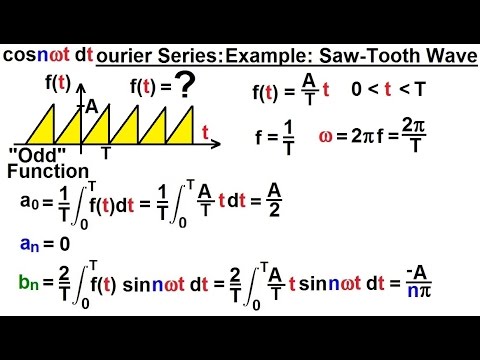
To determine the fourier coefficient a. Overview of fourier series the definition of fourier series and how it is an example of a trigonometric infinite series 2. We look at a spike a step function and a ramp and smoother functions too. Square waves 1 or 0 or 1 are great examples with delta functions in the derivative.

Half range fourier expansions. The even extension gives rise to a half range cosine series while the odd extension gives rise to a half range sine series. Fourier series of even and odd functions this section makes your life easier because it significantly cuts down the work 4. Even and odd functions.

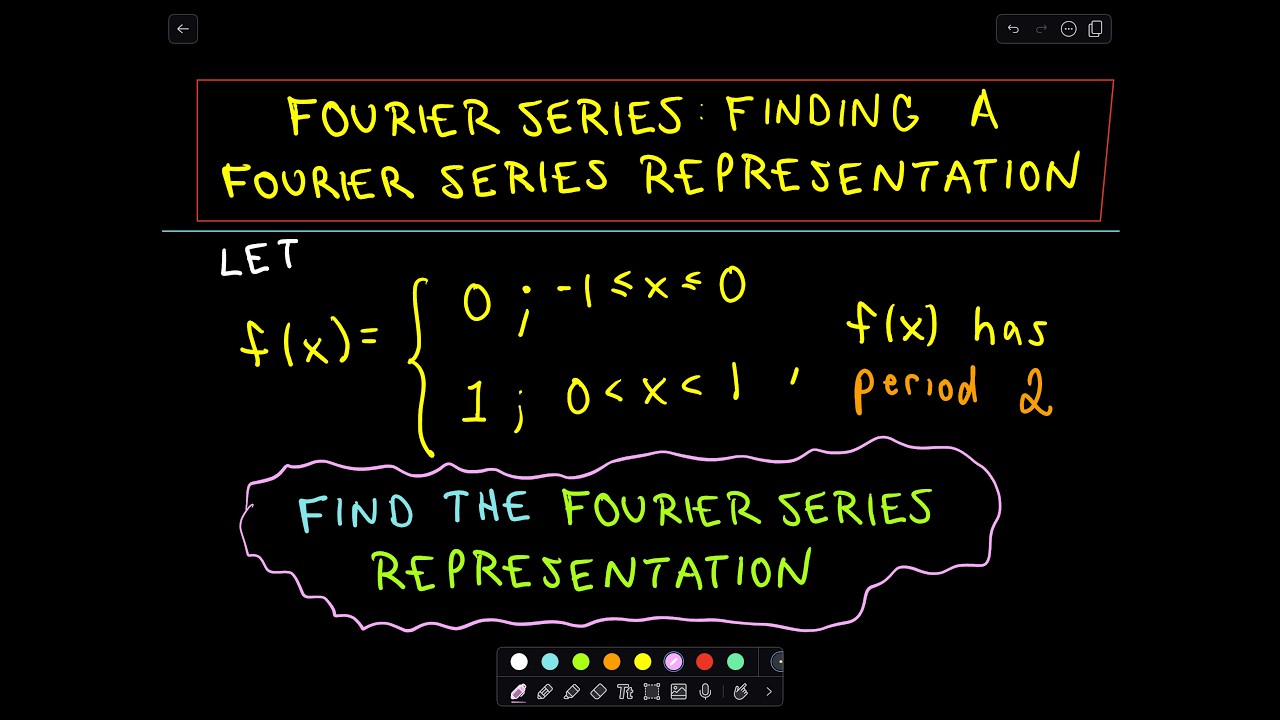
Fourier series of half range functions by smooth extension article pdf available in applied mathematical modelling 33 2 812 821 february 2009 with 1 728 reads how we measure reads. Sines cosines and exponentials eikx. 1 in a fourier series gives a series of constants that should equal f x 1. For example we might define f t 0 over π t 0.