Half Range Fourier Sine Series Formula
This allows the expansion of the function in a series solely of sines odd or cosines even.
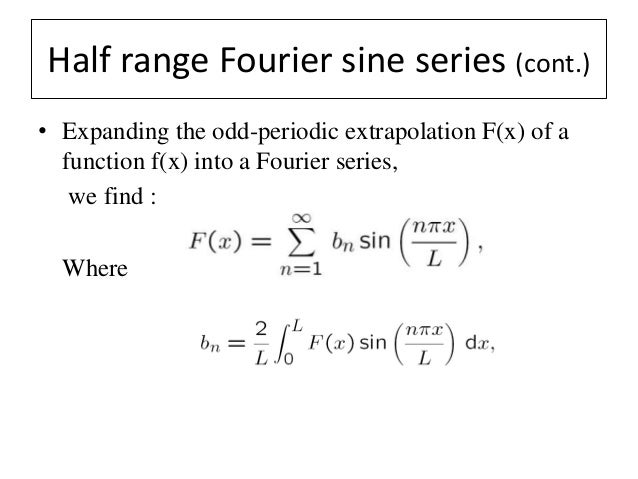
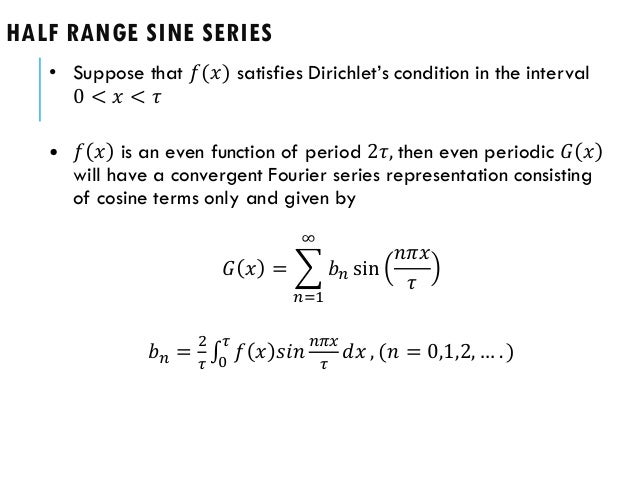
Half range fourier sine series formula. The fourier series of the half range even function is given by. F x a0 2 1 n 1 an cos nˇx l 14 12 an 2 l l 0 f x cos nˇx l dx. A half range fourier series is a fourier series defined on an interval instead of the more common with the implication that the analyzed function should be extended to as either an even f x f x or odd function f x f x. Sine series if f x is an odd function then the fourier half range sine series of f is defined to be displaystyle f x sum n 1 infty b n sin frac n pi x l which is just a form of complete fourier series with the only difference that.
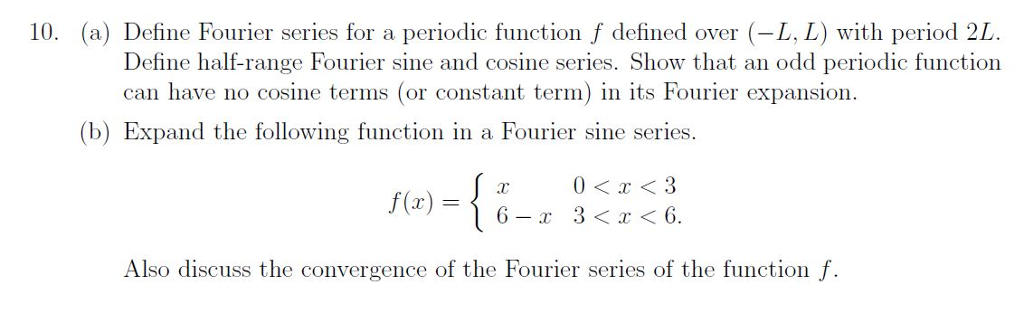
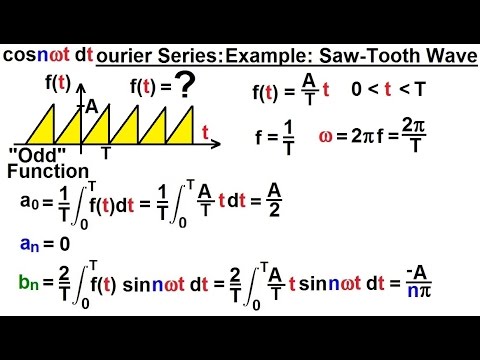
Find the fourier half range sine series and cosine series for f x x in the interval 0 p. 14 3 half range expansions if we are given a function f x on an interval 0 l and we want to represent f by a fourier series we have two choices a cosine series or a sine series. F x 1 n 1 bn sin nˇx l 14 14 bn 2 l l 0 f x sin nˇx l dx. F x x 0 x π 2 π x x p π 2.
Hence show that 1 1 2. Find the sine and cosine half range series for the function function.